Introducing the Fascinating World of Pentagons

Have you ever wondered about the intriguing properties of pentagons? These unique shapes with five sides and five vertices hold a plethora of secrets waiting to be discovered. In this article, we will delve into the theoretical background and explore the fascinating properties of regular pentagons. So fasten your seatbelt and get ready for a captivating journey!

Understanding the Basics: Definitions and Classification

Let's start with the basics. A pentagon can either be convex or concave, depending on the arrangement of its sides. Convex pentagons have all their interior angles measuring less than 180°, while concave pentagons have at least one interior angle larger than 180°. A regular pentagon is a special case where all sides and angles are equal, making it a symmetrical and harmonious shape. However, don't confuse it with an equilateral pentagon, which can be concave despite having equal sides.

Types of pentagons

The sum of the interior angles in a pentagon always adds up to a constant value of 540°, regardless of whether it is regular or irregular. To prove this, imagine dividing the pentagon into three non-overlapping triangles by drawing straight lines between its vertices. Each triangle has interior angles totaling 180°, so for three triangles, we have 3x180°=540°.

A pentagon can be divided into three triangles

Unveiling the Secrets of Regular Pentagons

Regular pentagons possess an array of intriguing properties that set them apart from other polygons. Let's explore a few of them:

Symmetry: A Gateway to Beauty

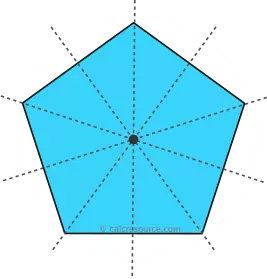
A regular pentagon boasts five axes of symmetry, each passing through a vertex and the midpoint of the opposite edge. All these axes intersect at the center of the pentagon, which coincides with its center of gravity or centroid.

Axes of symmetry of regular pentagon

Interior and Central Angles: The Art of Balance

In a regular pentagon, all interior angles are equal and measure 108°. The sum of these angles and the central angle (72°) is always 180°, making them supplementary. This symmetry is further highlighted when we divide the pentagon into five isosceles triangles, each with angles of 72°, 54°, and 54°.

Interior and central angle of a regular pentagon

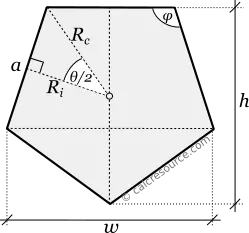
Circumcircle and Incircle: The Enigmatic Circles

A circumcircle can be drawn around a regular pentagon, passing through all five vertices and sharing the center with the pentagon itself. Similarly, an inscribed circle can be drawn, tangentially touching all five edges of the pentagon at their midpoints. These circles hold vital significance and contribute to the overall symmetry of the pentagon.

Circumcircle and incircle of a regular pentagon

Area and Perimeter: Measuring the Magic

To calculate the area of a regular pentagon, we divide it into five identical isosceles triangles. The area of each triangle is given by the formula A = 0.5aRi, where a is the side length of the pentagon and Ri is the inradius. Thus, the total area of the pentagon can be expressed as A = (5/2)a^2 or approximately 1.720a^2.

The perimeter of a regular pentagon is simply the sum of all its sides, which is 5a.

Unveiling the Bounding Box

The bounding box of a regular pentagon is the smallest rectangle that completely encloses the shape. Determining its exact dimensions requires a few calculations.

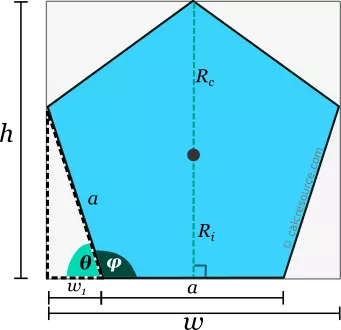
Height: A Delicate Balance

The height of a regular pentagon, represented by h, is the distance from one vertex to the opposite edge, passing through the center of the pentagon. It can be expressed in terms of the circumradius (Rc), inradius (Ri), or side length (a). Each of these expressions provides valuable insights into the height:

• h = Rc(1 + cos(theta/2))
• h = Ri(1 + (1/cos(theta/2)))
• h = (a/2)(1 + cos(theta/2)/sin(theta/2))

For theta = 72°, these equations simplify to approximate values: h ≈ 1.809Rc, h ≈ 2.236Ri, and h ≈ 1.539a.

Width: The Distance between Opposite Vertices

The width, denoted as w, is the distance between two opposite vertices of a regular pentagon. To determine this distance, we can utilize a right triangle formed by one side of the pentagon and the adjacent interior angle (varphi). By substituting theta = 72° and using basic trigonometry, we find that w ≈ 1.618a.

Drawing a Regular Pentagon

Would you like to draw a regular pentagon? It's easier than you think! Follow these simple steps:

1. Draw a line segment with a length equal to the desired side length of the pentagon.
2. Extend the line segment to the left.
3. Construct a circular arc with the center point at the right end of the line segment and a radius equal to the segment length.
4. Repeat step 3, but with the center point at the left end of the line segment. The radius remains the same.
5. Draw a line perpendicular to the line segment, passing through the intersection of the two arcs. This line should intersect the line segment at its midpoint.
6. Draw another line perpendicular to the line segment, passing through the left end of the line segment. Mark the intersection point with the circular arc drawn in step 4.
7. Draw another circular arc by placing the compass needle at the line segment's midpoint (found in step 5) and the drawing tip at the intersection marked in step 6. Rotate the compass until it crosses the extended line segment from step 2. Mark this new intersection.
8. Draw another circular arc by placing the compass needle at the right end of the line segment and the drawing tip at the intersection marked in step 7. Rotate the compass clockwise, marking two intersections—one with the arc drawn in step 4 and the other with the line from step 5. These two intersections represent two vertices of the pentagon.
9. Place the compass needle at the second intersection and the drawing tip at the first intersection (both marked in the previous step). Draw a circular arc that intersects the arc from step 3. The new intersection point is another vertex of the pentagon.
10. The two ends of the line segment, along with the three intersections marked in steps 8 and 9, form the five vertices of the regular pentagon. Connect these vertices with line segments to complete the shape.

Drawing a regular pentagon given its side length

It's worth noting that while the above procedure does not strictly adhere to a "ruler and compass" construction, it offers simplicity and reduces the number of steps required. If you prefer a strict geometric construction using only a ruler and compass, you can replace steps 5 and 6 with a traditional perpendicular line construction.

Wrapping Up

Regular pentagons are more than just shapes; they hold a captivating world of symmetry, angles, circles, and geometric wonders. Now that you've discovered the allure of this unique polygon, you can appreciate its beauty and explore further applications in the realms of mathematics, art, and design.

Regular Pentagon Cheat-Sheet

To assist you in your future adventures with regular pentagons, here's a handy cheat-sheet summarizing the main formulas and useful approximations:

• Rc ≈ 0.851a
• Ri ≈ 0.688a
• h ≈ 1.539a
• w ≈ 1.618a
• A ≈ 1.720a^2

We hope this article has shed light on the enchanting world of pentagons and inspired you to explore further. Enjoy your journey through the wondrous realm of geometry!